![]()
Carbon-14 Dating
Calculations
 Basic Radiocarbon Decay Rate Equation
Basic Radiocarbon Decay Rate Equation
If we represent the number of C14
atoms at any given time after the death of a plant or animal by N, and original
number of C14 atoms at the time of origin of the organism by No,
then the decay rate of C14
atoms is given mathematically as
follows:
N/No
= e-kt [1]
where t
is the elapsed time since the original living organism’s death in years, k is the decay constant which for carbon-14 has the value 0.0001209, and e is the number 2.71828…, the base number of natural logarithms.
This mathematical relationship graphs as follows:
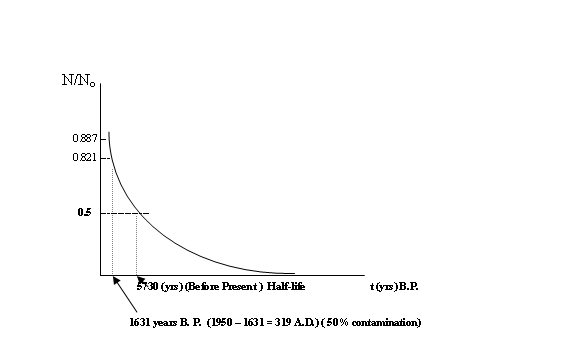
Radioactive decay rate for
Carbon-14
We see that the carbon-14 concentration
N/No decays to 0.5 ( i.e. 50%) after about 5730 years; this is
called the ‘half-life’ of carbon 14.
Example 1: No contamination
If we assume, for example, that the true
known age of a hypothetical linen test material is 1 A.D., i.e. 1999 years
before, say, 2000 A.D., then the value of N/No = e-.0001209 x
1999 = 0.785. That is to say,
78.5% of the original carbon 14 atoms present at 1 A.D. still remain, and 21.5% have decayed, over the 1999 years since
the flax plants from which the linen was woven were harvested.
Therefore, in running a radiocarbon dating
test on the sample, we would expect to get an instrumental reading
corresponding to a ‘radiocarbon age ‘t’
of 1999 years [1].
This would yield an historical age for the
sample of 2000 – ‘t’ = 2000 – 1999 = 1 A.D. in agreement with what we would
already have known for the example.
Example 2: 100% modern contamination
Weight of clean linen sample: 10
milligrams. Origin of linen sample
taken as being 1 A.D.,
Assume a weight of olive oil contamination
of 10 milligrams (added, say, at the time of the radiocarbon
test by mistake). Known age of olive oil
contamination: 1 year.
Total sample weight is now 20 mg.
Value of N/No for clean
linen sample ( t = 1999 yrs.) = e-.0001209
x 1999 = o.785 as before.
Value of N/No for olive oil ( t
=1 yr.) = e-.0001209 x 1 =
0.999879
Combined N/No for contaminated
sample = 10/20 x 0785 + 10/20 x 0.999879 = 0.892
Inserting this value for N/N0
in Eqn. 1 and solving we get the experimental radiocarbon age ‘t’ to be expected as:
t =
ln[0.892 ]/ -.0001209 = 945 ‘years’
The calculated ‘historical age’ for the
sample then (again assuming we are in the year 2000 A.D.) is 2000 – ‘t’ = 2000
-945 = 1055 A.D.
So we see that the effect of 100%
contamination with modern carbon ( age 1 year) would be to artificially advance
the historical date for the linen
forward from a true age of 1 A.D. to 1055
A.D., that is by over a thousand years.
Exampe 3: 100% contamination of average date 1000 A.D.
Weight of clean linen sample 10 mg. Known
age of sample 1 A.D..
Weight of contamination 10 mg. Assumed
average or mean age of the olive oil contamination is now taken as 1000 A.D.
Value of N/No for clean
sample: 0.785
Value of N/No for olive oil of
age 1000 A.D. = e -0.0001209 x 1000
= 0.886
Combined N/No for contaminated
sample = 10/20 x 0.785 + 10/20 x 0.886 = 0.8355
Radiocarbon age ‘t’ to be expected = ln
[0.8355.] /-.0001209 = 1487 yrs B.P.
Historical age of linen sample = 2000 –
1487 = 513 A.D.
Therefore the experimentally obtained
radiocarbon result for a test sample of known origin of 1 A.D. which has
been contaminated with 100% of carbon of
average age of 1000 A.D. will give an apparent computed historical date of
origin of 513A.D. instead of the correct age of 1 A.D.
From these three examples we see that when radiocarbon
tests are run two questions must be
asked and answered.
First, Is
the sample clean? If so then the
historical age is simply [Year of test]
minus[the number of radiocarbon years
“t” before the year of the test].
Second. If
the sample is contaminated, can it be cleaned? If it cannot be cleaned then the non-removable
contamination must be taken into account in the calculation of the historical
age as in example 3 above..
Were the Shroud test samples
contaminated? How to correct for contamination
The
unit area weight (specific weight, weight per square centimeter) of the 1988
test samples cut from the corner of the Shroud is simply the weight of each
sample divided by its area. If this unit weight is found to be around 23 mg/sq
cm, which is the known average unit area
weight for the Shroud as a whole, then the sample is clean and
representative. If it is more than 23
mg/sq cm, then the sample is
contaminated and non-representative.
The
test samples in the 1989 report in Nature [2] each weighed
approximately 50 milligrams, which
agrees with the official figure given by Testore who weighed the samples in
1988 in Turin. The area of each samples
was about 1.166 sq. cm each, as given
from the data of Moretto [3], former Secretary to
the International Centre of Sindonology
of Turin and Secretary of the Journal Sindon
:
“The sample (was) reduced to 7 x 1 cm after removal of
the frayed bits round the edges. This was then divided into two roughly equal
parts, one of which was retained, and the other subdivided into three . Each of
the three laboratories ...was allocated a
little more than a square centimeter of the Shroud textile, for this to
undergo dating by the carbon 14 method.”

Thus
the 7 x 1 cm trimmed strip was first cut
in half , and then three equal
samples were cut from this one-half piece. This makes each of the three samples
1/3 of 3.5 sq. cm or 1.166 sq. cm. in area ( i.e. “ a little more than a square
centimeter” as stated by Moretto.).
The unit area weight of each sample is then
calculated as its weight, 50 mg, divided by its area of 1.166 sq.cm for 42.9
mg.per sq. cm. ( 50/1.166 = 42.9).
Since the average weight for the Shroud as a whole, away from any
heavily handled corner, is only 23 mg per sq., we have a ratio
of contamination 42.9/23 or 1.87
i.e. 87%. This is not at all surprising, since the
samples were cut from a heavily handled corner which even shows up visually as
a slightly stained or darker area on photographs of the Shroud.
(Experimental
tests have also been run to determine
the amount of extra weight that is taken up from human fingerprints in
handling an object. The results easily reproduce the 87% level of contamination on the samples
cut from the corner of the Shroud)
How to correct for the 87%
contamination to get the valid estimate
of the historical age of the Shroud
Given
the 87% contamination of the
samples, the task for the radiocarbon
laboratory is then to determine if it is removable or not. Here the Nature
Report is ambiguous. The authors say only that the
samples were vigorously cleaned and that the Zurich sample showed “no evidence
of contamination” after the cleaning.
This could, of course, mean either that the contamination could not be
removed by cleaning or, alternatively, that there was none. But, since we already know that it was 87% contaminated because of the very
high unit area weight of 42.9 mg. per
sq. cm., the only conclusion is that the
contamination was totally non-removable.
Later,
however, Prof. E. T. Hall of the Oxford
radiocarbon team and himself one of the Nature
authors, increased the ambiguity by stating in conversation that all samples
lost about 20% of their weight in the cleaning! This weight loss could, of course, be partly a loss of
contaminating carbon and partly a loss of some linen fibres from abrasion.
However, let us conservatively assume that the 20% weight loss was all due to
contamination, and reduce the non-removable
carbon contamination ( on all samples, including Zurich) by Hall’s full 20% from 87% to 50% ( 0.8 x 1.87 = 1.496 = 1.50) The radiocarbon age must then be
adjusted, to account for this 50% contamination by more recent carbon picked up
from human handling at the corner of the Shroud from which the samples were
cut, in order to estimate the true
historical date for the origin of the linen.
If
we estimate that the average date of origin of the 50% carbon contamination is,
say, 1000 A.D , then the necessary adjustment to the ‘radiocarbon age’ t of 691 years reported in
Nature is as follows:
Specific
weight of each sample 42.9 milligram per sq.cm.
Less
20% loss on cleaning -
8.6 mg
Weight
of clean linen plus non-removable carbon contamination 34.3 mg
Specific
weight of clean linen of Shroud 23.0 “
Net
contamination 11.3 “
N/No
for the clean linen ( t = 1988-33=1955 years) = e-0.0001209 x 1955 =
0.789
N/No
for contamination (t = 1988-1000 = 988) = e-.0001209 x 988 = 0.887
Correction
to t of ‘691 years’ for contamination ( N/No = 0.887 ) is:
N/No (comb.) = { fa
x 0.789} + { fb x 0.887}
=
{ 23/34.3 x 0.789} + { 11.3/34.3
x 0.887}
= 0.529 +
0.292 = 0.821
t (combined) =
loge 0.821/-0.0001209 = 1631 years ( instead of 691 yrs).
The
corresponding estimated historical date, corrected for the contamination, is
just 1988-1631 = 357 A.D. , or 1950-1631 = 319
A.D if we take the base year as 1950 instead of the actual year of the
test).
To
sum up, contamination was not considered in the Nature report, whose authors
simply went ahead as though the samples were clean and published their
erroneous mediaeval date for the origin
of the linen of the Shroud [1988-691= 1297
or 1950- 691 = 1259 A.D.,
declaring that they had 95% confidence in their calculation. Quite naturally,
this assertion has unraveled over the
years, after the official Turin information on the sample sizes which showed
the ignored contamination, become widely available. .
Further correction for C-14 enhancement by neutron flux, if
any: Finally, we should account for the enhancement of C14 by any possible neutron flux.
The exchange between Phillips and
Hedges in Nature n 1989 [4] generated widespread interest and discussion. One
objection raised to the C14 enhancement by neutron flow was that,
while neutrons would undoubtedly enrich the C14 content of linen,
this was no proof that the new C14 atoms so produced would remain in
the linen and not just simply diffuse out and evaporate into the air.
J-B. Rinaudo [5] settled this point experimentally by
irradiating a piece of ancient linen of
known historical age with a neutron flow in a reactor, and then
measuring the radiocarbon age. He found, as predicted, that the apparent age of
the cloth had been greatly advanced by the neutrons in accordance with the
predictions, thus proving that the new C14 atoms produced by the
neutron flow did indeed bind to the linen and remained there to alter the
radiocarbon date. Whether this result will eventually prove relevant to the
Shroud mystery will depend on whether a plausible physical source for the
postulated neutron flux can be established. This will be examined in further
Updates to the website.
A final word of caution to the non-scientist
here. One must be careful in
interpreting scientific statements about radiocarbon dates since the
measurements and calculations are not an
exact historical accounting or balancing procedure . The Nature
authors for example, quite properly rounded all their date estimates only
to the nearest 10 years. Exact years
here are only misleading. In any case, we are only concerned with the broad
question of whether the Shroud is
medieval or ancient. Therefore, the minor complications of such things as
the 38 years difference between “years B
P, meaning “years before 1950” on the
one hand and the “years before 1988 the
date of the test measurements ´on the other hand are of no importance to the
conclusions. For another example, the Nature
report gives the mean radiocarbon date of 691 BP plus or minus 31. This gives
an historical age slightly different from their statistical average age of
around 1325 A.D. but there is no essential contradiction. The critical analysis
given here is not concerned with minor
arithmetical, statistical or calibration details, or with irrelevant slight
inconsistencies. It deals with the major
non-removable contamination of the Nature
samples which was neither taken into
account nor reported.
References
1. For historical reasons, it has become customary in
radiocarbon work to take B.P. ( “before
present” ) to mean “before 1950 A.D.”, in spite of the fact that tests made today
are some 50-odd years later. This small
technical correction to the calculation
of dates is not made here for reasons of
simplicity and clarity of presentation to non-scientist readers. To take it
into account for the Shroud tests in
1988 would mean an adjustment to calculated dates of only 38 years ( 1988-1950 = 38).
It should also be noted that professional
Carbon-14 dating calculations are very
sophisticated and specialized; the calculations given here are simplified for
clarity, but with no loss of validity.
2. P.E. Damon, et al., “Radiocarbon dating
of the Shroud of Turin”, Nature, 337, 6208, pp 611-615, Sept. 11, 1989.
3. Gino Moretto, The
Shroud: A Guide ( English transl.).
Paulist Press, New York, N.Y. 1996.
4. T.J. Phillips and R.E.M. Hedges.
Correspondence in Nature, 337, 16 February, p. 594. 1989.
--------- T.J. Phillips, Reply to Dr. R.E.M Hedges’ Nature correspondence. British Society
for the Shroud of Turin Newsletter No. 22, May 1989, pp. 8-11.
5. J. B. Rinaudo, “Image formation on the Shroud of Turin explained by a protonic
model affecting radiocarbon dating” III
Congresso internazionale di studi sulla Sindone, Torino, 5-7 Giugno 1998.
---------------------, “Theory No. 3: French Scientist
Jean-Baptiste Rinaudo”. British Society
for the Shroud of

![]() MAIN PAGE / The Historical Facts / The Scientific Facts / Other Shroud Sites / Carbon 14 dating in 1988
MAIN PAGE / The Historical Facts / The Scientific Facts / Other Shroud Sites / Carbon 14 dating in 1988
Copyright © 2004. Bernard A. Power